A few weeks ago I asked Twitter what math topic they thought could use a game.
@NoraOswald my most hated topic to teach: absolute value inequalities. Betcha can't make that fun.— Susan Russo (@Dsrussosusan) June 25, 2015
Challenge accepted! I do have an Absolute Value EQUATION game (click here to read about that) but she is asking for inequalities.
Since I'm at home creating games rather than my classroom, I do not have access to a large white board. You'll have to use your imagination with my photos about playing this with a whole class.
2 - 5 Players or Teams
Algebra 1 Students
After learning about Absolute Value Equations and Inequalities.
BUT....you could use this game leading from student Abs Val Equations to Inequalities
Classroom with a large magnetic whiteboard.
Because you want your students to love math.
Game Objective:
Work together as a class to get the highest score possible.
Materials:
A standard deck of cards with all the face cards removed (Jacks, Queens, and Kings)
Two arrows. On one side you want an open circle and on the other side a filled in circle.
You will need magnets on both sides.
You'll need to keep track of the classes score. I recommend posting the table of possible points for all groups to see. The class starts with 6 points.
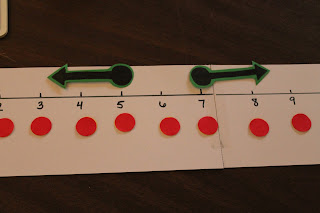
You will need to draw a number line from -20 to 20.
You will need 32 2-sided circles (or squares, or stars, or whatever shape you want). Hint: squares are easier to cut. My circles are yellow on one side and red on the other. I used a 3/4 inch hole punch for each of the colors and then glued them together. However, your circles will need to be much larger if you are playing with the whole class. Place magnets on both sides of the circles.
Set Up:
Place one circle at each integer on the number line from -15 to 15. There will be one circle remaining. Use that circle to keep track of what color you are working on.
 |
| The 'extra' circle lets players know that they are trying to make all the circle red. |
Deal 5 cards to each team.
Game Play:
On a teams turn, they will create an Absolute Value Inequality using at least 2 of their cards. Teams may choose to use a plus or minus sign. They may also decide which of the 4 inequalities they want to use. (<, <=, >, >=)
Once they create their inequality, they place the arrows to match their inequality. All the circles that are within the domain of the inequality are flipped to the other side and showing the other color.
The team hands in their 2 cards and receive 2 new cards from the deck.
Once there are only a few of the unwanted color remaining, points are given as follows.
4 circles remaining: 1 point
3 circles remaining: 2 points
2 circles remaining: 3 points
1 circle remaining: 4 points
0 circles remaining: 5 points
If a team is able to get the unwanted color down to say 4, then next team can decide if they can keep going to add more points, or try to flip the circle back to the other color.
At the end of class, see how many points are accumulated and try to beat that score next time. Or try to beat another class. Or keep track and beat that score next year.
** Teams are able to add the cards together to get a required higher number. Each extra card used will cost 1 point. All cards will be replaced at the end of the round so that each team still has 5 cards.
Once they create their inequality, they place the arrows to match their inequality. All the circles that are within the domain of the inequality are flipped to the other side and showing the other color.
The team hands in their 2 cards and receive 2 new cards from the deck.
Once there are only a few of the unwanted color remaining, points are given as follows.
4 circles remaining: 1 point
3 circles remaining: 2 points
2 circles remaining: 3 points
1 circle remaining: 4 points
0 circles remaining: 5 points
If a team is able to get the unwanted color down to say 4, then next team can decide if they can keep going to add more points, or try to flip the circle back to the other color.
At the end of class, see how many points are accumulated and try to beat that score next time. Or try to beat another class. Or keep track and beat that score next year.
** Teams are able to add the cards together to get a required higher number. Each extra card used will cost 1 point. All cards will be replaced at the end of the round so that each team still has 5 cards.
Some Examples of Game Play:
Here are the five cards that team 1 has.
They decide to use 10 and 2 to create the following inequality.
They position the arrows. Positive 10 is the 'middle' and 2 is the distance. They picked <, so the arrows will points toward the middle.
I like to remind the students that since the distance is less than 2, they can't flip circles that are farther away than 2.
They flip over the circle in that domain.
Since there are more than 4 yellow circles showing (the unwanted color), no points are awarded.
Team 2 has the following cards.
They decide to use 6 and 2 to create their inequality.
The arrows...
Flip the circles...
Back to team 1...
The inequality...
The arrows....
Flip the circles...
Back to team 2...
They are going to use 3 cards to create their inequality. I will cost them 1 point for each extra card used.
The class point total goes down to 5 since an extra card is used.
Place the arrows...
Flip the circles...
Internal thought (okay, someone should have made some points by now). Anyway back to player 1.
The inequality...
The arrows...
The flip...
At last! We are down to 4 yellow circles, and the class has scored 1 point. If the next team is able to flip over some of those yellow, the class could earn even more points. If not, we flip the 'extra' circle to show that we are now trying to make them all yellow.
And here is the point we earned! We went from 5 to 6.
Here is another example, because I noticed that I didn't use > in the above example.
We are trying to make the red circles yellow.
The 5 cards...
D'oh! I forgot to take a photo of the inequality. Let's see, it looks like |x-6| >= 1
The arrows...
The flip. This was great because I was able to flip over all the circles except for 1 and the class scored 4 points. The next team is unable to flip that last circle over, so now we are trying to make them all red.
The cards...
The inequality...
The arrows...
The flip...
I guess you get the idea.
Other Thoughts:
You might be wondering why the circles aren't placed to the end of the number line. I was play-testing it that way, but it was difficult to flip the circles between 16 and 20 (and -16 and -20). Even with the whole adding-two-cards-together rule, it wasn't easy to get those high numbers needed. The arrows can still be placed from 16 to 20 (and -16 to 20).
I did play-test this as a competitive game, but it's too easy to sabotage the board so that no one gets points. I thought working together was the best way.
This can be a tabletop game for your students as well. Instead of each class trying to get the highest score, each group of students playing could be trying to get the highest score.
I wish I had access to some students, but I'll have to wait and see if this is even fun when school is back in session. Anyway, this gives me plenty of time to keep tweaking and get your ideas...which are appreciated.



































Was thinking about this my whole walk. (Except for during Tai Chi.) Love the mechanic, with the cards and the structure and the line. Was wondering about more interaction. Two ideas, neither tested:
ReplyDelete1) Both teams get three or four cards, make an inequality face down, reveal. Your score = to the length you covered that the other team didn't. I.e. |x-3|<7 vs |x- -1|<4,
the 3,7 team covered -4 to 10,
-1,4 team covered -5 to 3.
So 3,7 scores [3,10) 7 points. -1,4 scores (-5,-4] 1 point.
2) Each team draws 4 cards. Target team sets three points to capture, -5, 2, 12.
Hunter team gets the leftover card and forms |x-5|<8, scores 2 points for capturing 2 & 12.
Just spitballing!
Tried a simplified version of this in summer school today and it went great... Thanks so much! I had five pairs of kids-- each pair got three cards and a whiteboard. I gave them about 4 minutes to come up with their best solution and then we debriefed/compared to find the winner. We did a few rounds and the kids really enjoyed it and they were able to deepen their knowledge quite a bit as they developed a strategy. Really cool idea-- I've already shared with my math peeps. Thanks again.
ReplyDelete